Ah ! Got it.
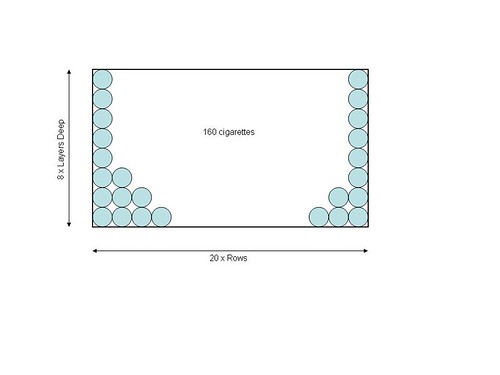
My response to HH explains why I only drew 25 cigarettes - it was lazyness on my part. I had hoped the "160 cigarettes" caption made it clear how many cigarettes were initially packed into the carton.
One of these days I might get around to looking at 19 cigarettes on the bottom row, 18 in the next layer, then 19 etc thus giving xxx, which might, or might not fit and might or might not be more than 176........one of these days............
Meanwhile, 176 is as good as I've seen, and with nice arithmetic.
Cheers
Don